Can Standard Deviation Be 0

A standard deviation is a number that tells united states of america
to what extent a set of numbers lie apart. A standard deviation can range from 0 to infinity. A standard departure of 0 ways that a list of numbers are all equal -they don't lie apart to whatsoever extent at all.

Standard Departure - Instance

Five applicants took an IQ test as part of a task application. Their scores on iii IQ components are shown beneath.

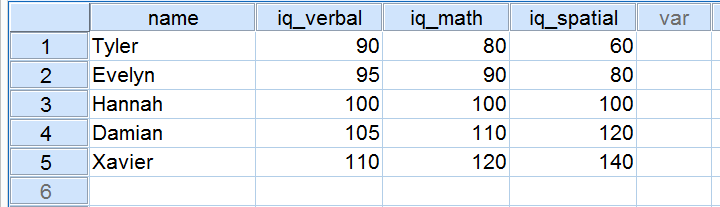
Standard Deviation - Screenshot Raw Data Points

Now, permit's take a close look at the scores on the three IQ components. Note that all three have a mean of 100 over our 5 applicants. However, the scores on iq_verbal lie closer together than the scores on iq_math. Furthermore, the scores on iq_spatial prevarication further apart than the scores on the outset ii components. The precise extent to which a number of scores lie apart can exist expressed as a number. This number is known as the standard departure.

Standard Deviation - Results

In real life, we obviously don't visually audit raw scores in order to see how far they lie autonomously. Instead, we'll simply have some software calculate them for u.s.a. (more on that after). The table beneath shows the standard deviations and another statistics for our IQ information. Note that the standard deviations confirm the pattern we saw in the raw data.

Standard Deviation in Descriptive Statistics Table

Standard Deviation and Histogram

Right, let'south make things a flake more visual. The figure beneath shows the standard deviations and the histograms for our IQ scores. Note that each bar represents the score of ane applicant on ane IQ component. Once once more, we see that the standard deviations indicate the extent to which the scores lie autonomously.

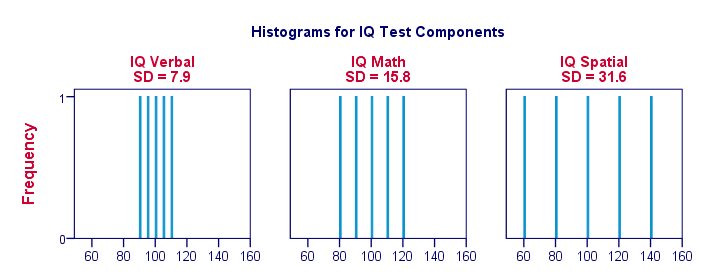
Standard Deviation - More than Histograms

When we visualize data on only a handful of observations as in the previous figure, we easily run into a clear picture. For a more realistic case, we'll present histograms for one,000 observations below. Importantly, these histograms have identical scales; for each histogram, one centimeter on the x-axis corresponds to some forty 'IQ component points'.

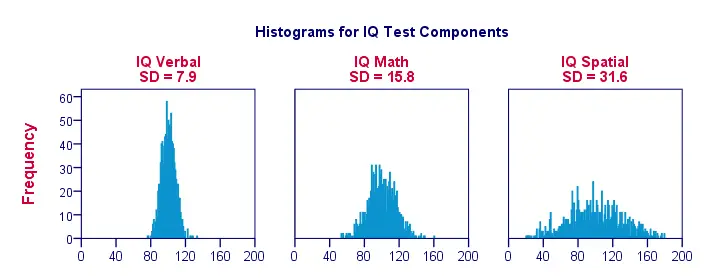
Notation how the histograms allow for rough estimates of standard deviations. 'Wider' histograms point larger standard deviations; the scores (x-axis) prevarication further apart. Since all histograms have identical surface areas (corresponding to 1,000 observations), higher standard deviations are likewise associated with 'lower' histograms.

Standard Difference - Population Formula

Then how does your software calculate standard deviations? Well, the basic formula is

$$\sigma = \sqrt{\frac{\sum(X - \mu)^2}{Due north}}$$

where

$10$ denotes each split up number;
$\mu$ denotes the mean over all numbers and
$\sum$ denotes a sum.

In words, the standard difference is the square root of the boilerplate squared difference between each private number and the mean of these numbers.

Importantly, this formula assumes that your data contain the entire population of involvement (hence "population formula"). If your data incorporate merely a sample from your target population, see beneath.

Population Formula - Software

You can utilise this formula in Google sheets, OpenOffice and Excel by typing =STDEVP(...) into a cell. Specify the numbers over which yous want the standard departure betwixt the parentheses and press Enter. The figure beneath illustrates the idea.

Oddly, the population standard divergence formula does not seem to exist in SPSS.

Standard Deviation - Sample Formula

Now for something challenging: if your data are (approximately) a simple random sample from some (much) larger population, then the previous formula will systematically underestimate the standard deviation in this population. An unbiased figurer for the population standard deviation is obtained by using

$$S_x = \sqrt{\frac{\sum(Ten - \overline{X})^2}{Northward -1}}$$

Regarding calculations, the big departure with the get-go formula is that nosotros divide by $n -i$ instead of $n$. Dividing by a smaller number results in a (slightly) larger result. This precisely compensates for the aforementioned underestimation. For big sample sizes, however, the ii formulas have virtually identical outcomes.
In GoogleSheets, Open up Office and MS Excel, the STDEV function uses this second formula. It is also the (only) standard divergence formula implemented in SPSS.

Standard Divergence and Variance

A second number that expresses how far a set of numbers lie apart is the variance. The variance is the squared standard divergence. This implies that, similarly to the standard deviation, the variance has a population besides as a sample formula.
In principle, it's awkward that two dissimilar statistics basically express the aforementioned property of a set of numbers. Why don't we just discard the variance in favor of the standard deviation (or reversely)? The basic reply is that the standard deviation has more desirable properties in some situations and the variance in others.